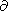 /
/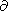 t
t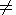 0. So, for a clear understanding
of the physics of the process, in particular from the energetic
point of view, it is more accurate to use a time-varying rate.
0. So, for a clear understanding
of the physics of the process, in particular from the energetic
point of view, it is more accurate to use a time-varying rate.
N. N. Volkonskaya, T. N. Volkonskaya, and V. S. Semenov
Institute of Physics, St. Petersburg University, St. Petersburg, Russia
Helfried K. Biernat
Space Research Institute, Austrian Academy of Sciences, Graz, Austria
Many processes observed inside the magnetosphere of Earth and other planets, and on Sun and other stars are associated with conversion of magnetic energy. There are many experimental results, which demonstrate that the processes of conversion of magnetic energy to kinetic and internal energy of the plasma occur in various situation [Coroniti, 1985; Hones, 1984]. For example, these phenomena take place during magnetosphere substorms in the magnetotail, at the magnetopause near the solar point (for a south direction of the IMF) and in the cusp region (for a north direction of the IMF), during solar flares and flares of some types of stars. It means that we deal with a fundamental physical process in the plasma. At present, there are several theories which describe the conversion of magnetic energy to kinetic energy of plasma. Most famous models are the pure resistive magnetic field Sweet-Parker dissipation [Parker, 1963; Sweet, 1958] tearing mode instability [Furth et al., 1963; Ottaviani and Porcell, 1995] and Petschek [1964] reconnection.
The model of reconnection suggested by Petschek [1964] explains the fast energy release in solar flares. Magnetic field dissipation needs only be present within a small region known as the diffusion region, and the energy conversion occurs primarily across nonlinear waves, or shocks in a highly conducting plasma.
Most of the
used
analytical models
describe the steady-state
regime of reconnection
[Priest, 1985],
but in nature we usually
deal with explosive-like phenomena, where
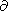 /
/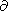 t
t 0. So, for a clear understanding
of the physics of the process, in particular from the energetic
point of view, it is more accurate to use a time-varying rate.
0. So, for a clear understanding
of the physics of the process, in particular from the energetic
point of view, it is more accurate to use a time-varying rate.
Therefore Petschek's idea has been elaborated by Semenov et al. [1985], Biernat et al. [1987], and Heyn and Semenov [1996] for time-dependent conditions. There are a number of aspects of time-dependent reconnection which have no analogous behavior to the steady-state case [Biernat, 1993; Semenov et al., 1992]. The consideration of a time-varying reconnection rate makes possible the detailed analysis of the process of energy conversion and the change of momentum and energy of the plasma during the pulse of reconnection.

|
| Figure 1 |
However, reconnection is a rather complicated process. It will be shown that besides the plasma jets, a special MHD wave is generated in the inflow region and propagates with the jets. This wave contains the amount of energy which is equal to twice of the kinetic energy of the accelerated plasma inside the FRR. A complex MHD object which consist of an outflow region and a special MHD wave is formed and transports both kinetic and magnetic energy but, as will be shown, no momentum.
The reconnection problem for time-varying conditions is solved for the case of weak reconnection which implies that the dissipative electric field must be much less than the Alfvén electric field:
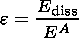 | (1) |
At present it is not exactly known what kind of plasma instability is responsible for the generation of the reconnection electric field. Therefore it is convenient to prescribe the behavior of the electric field E diss along a reconnection line a priori as a boundary condition rather than to calculate it from plasma theory.
The solution of the reconnection problem in an incompressible plasma in dimensionless form for the 2D symmetric configuration of magnetic field can be present as follows [Biernat et al., 1987; Semenov et al., 1985]
 | (2) |
 | (3) |
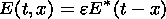 | (4) |
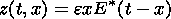 | (5) |
where equation (2) are the components of the magnetic field, equation (3) is the velocity of the plasma, equation (4) is the electric field inside FRR, equation (5) is the shape of the shock wave and E* is the electric field which is normalized to the maximum value of the dissipative electric field E diss, so the E* max=1.
This solution in the outflow region does not look much more complicated than the original Petschek solution and in fact it can be reduced to the latter by the substitution E*= const. Hence we can expect that the energy balance of the time-dependent reconnection might be very similar to the steady-state regime inside the outflow region. The main difference between steady-state and time-dependent conditions can bee seen in the inflow region where the magnetic field disturbances begin to play an important role:
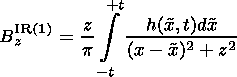 | (6) |
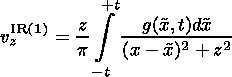 | (7) |
 | (8) |
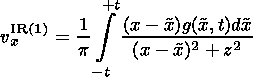 | (9) |
The expressions for the first-order component of the magnetic field and the velocities of the plasma in the inflow region above and below current sheet are obtained from the Poisson integrals. Here h(x,t) is the first-order Bz component at the boundary of the outflow region and g(x,t) is the first-order vz component at the same boundary, which is derived from the condition of the continuity of the normal component of the magnetic field Bn=0 and of the normal component of the velocity vn - Dn=0, where Dn is the velocity of the shock:
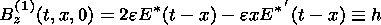 | (10) |
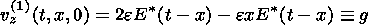 | (11) |
All quantities are normalized to a magnetic field strength B0, the velocity vA=B0/4pr, the characteristic duration of the reconnection pulse T0, the length vAT0, and the energy density B02/8p. Because of symmetry, it is sufficient to consider all equations in the first quadrant only.
In the immediate vicinity of the reconnection line,
x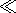 t,z
t,z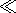 t, during the switch-on phase, this solution can be reduced to
the well-known Petschek solution. The time-dependent solution is
not much more complicated, but it can describe much more physics.
t, during the switch-on phase, this solution can be reduced to
the well-known Petschek solution. The time-dependent solution is
not much more complicated, but it can describe much more physics.
Different reconnection electric fields can be investigated in the framework of the time-dependent reconnection theory, such as burst-like, impulsive quasi-steady-state, etc. We will concentrate to the case of burst reconnection.
During the reconnection process, plasma is highly accelerated at the slow shocks and collected inside the FRRs so that the magnetic energy is converted into kinetic energy of the plasma. Taking into account that the kinetic energy density rv2/2 is normalized to the magnetic energy density B02/8p and in the dimensionless form is just vv2, we calculate the kinetic energy of the plasma in the FR region.
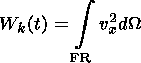 | (12) |
where dW is the element of the volume.
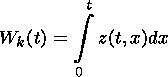 |
 | (13) |
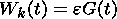 | (14) |
where G(t) is the volume of the outflow region and F(t) is the reconnected magnetic flux:
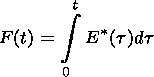 | (15) |
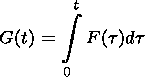 | (16) |
Moreover, the FRR transports energy, mass, and momentum. The momentum of the accelerated plasma inside the FRR in dimensionless form can be calculated in a similar way:
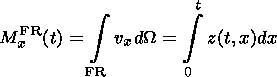 | (17) |
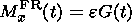 | (18) |
All results are obtained for an arbitrary reconnection rate and because of that they are quite general, but to illustrate our conclusions, we model a pulse of reconnection by the following dissipative electric field:
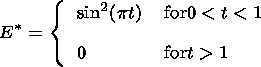 | (19) |
|
| Figure 2 |
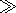 1 is asymptotically
1 is asymptotically
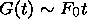 | (20) |
where F0 is the reconnected flux during the whole pulse of reconnection. Therefore G(t) is proportional to the distance from the former site of reconnection.
The total change of magnetic energy inside the FRR in dimensionless form is simply equal to
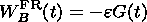 | (21) |
So the kinetic energy of the plasma is equal to the decrease of energy of the magnetic field inside FRR. Thus, the magnetic field inside the FR region effectively disappears and the energy of the magnetic field in the outflow region is directly transformed into kinetic energy of the accelerated plasma so that the energy density in the FRR is conserved at each moment of time in every point.
It should be noted that this result follows just from the simple relation
 | (22) |
which corresponds to the energy balance in the steady-state case.
Thus, the time-dependent regime of reconnection inside the outflow region is rather similar to the steady-state one. Magnetic energy is converted into kinetic energy with the efficiency coefficient to be equal to one (equation (22)). The only important difference between these two regimes is the switch-off phase which is essentially unsteady.
Perturbation of the magnetic field and velocity in the inflow region are small (in first order with respect to e ), and therefore it can be supposed that the total energy of magnetic disturbances in the IR is also rather small. However, we have to take into account that although the inflow region is much less disturbed compared to the FRR, a bigger area is affected. Hence we have to make a more detailed analysis.
By definition, the total change of energy of the magnetic field in the inflow region is equal to
 | (23) |
It is advantageous to introduce a vector potential A so that
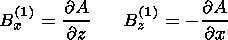 | (24) |
with the gauge condition
A(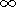 )=0. Now we can integrate along
the
z axis in equation (23) to obtain
)=0. Now we can integrate along
the
z axis in equation (23) to obtain
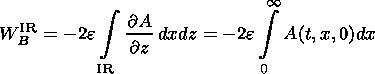 | (25) |
Taking into account that the first-order component of the magnetic field at the boundary of the FRR is sufficiently simple (equation (10)), we can calculate the magnetic potential at the x axis:
 | (26) |
Last, we obtain the following expression for the whole change of the magnetic energy in the inflow region:
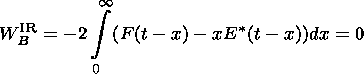 | (27) |
The total change of the energy of the magnetic field in the IR turns out to be exactly zero. Hence it would be expected that the contribution of the magnetic disturbances to the energy balance is negligibly small in the inflow region, and energy conversion takes place only at shocks. However, in principal, there is still the possibility that positive and negative disturbances can compensate each other. Thus, it is necessary to analyze the behavior of the density of the magnetic field energy in different parts of the inflow region and to find the details of the energy distribution. By the variation of the limits of the integral (27) we can obtain the values of the change of the magnetic field energy at an arbitrary part of the IR.
The energy of magnetic disturbances in a column
(x:[x,x+dx];z:[0,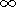 ))
is simply equal to (see
equations (25) and (26))
))
is simply equal to (see
equations (25) and (26))
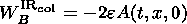 |
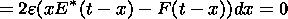 | (28) |

|
| Figure 3 |
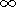 )):
)):
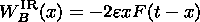 | (29) |
This function linearly decreases from zero at the former site of reconnection to some minimum negative value near the position of the FRR and then increases to zero. This implies that the magnetic energy is negative for the wake of the field reversal regions and positive exactly in the column above the shocks. It should be emphasized that these positive and negative energies exactly compensate each other (see Figure 3).
From the physical point of view we can interpret this effect as follows. Fast moving shocks compress the magnetic field near their leading fronts and produce a compression wave. To some extent this is similar to the generation of a head wave from a fast moving boat on the water. Behind the moving FR regions the magnetic field becomes weaker than before reconnection, which leads to decrease of magnetic energy in the wake.
It can be shown that the amount of the positive energy in the compression wave is equal to
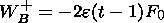 | (30) |
Asymptotically, for
t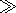 1, the maximum value of this positive
pulse is
1, the maximum value of this positive
pulse is
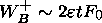 | (31) |
which is double the kinetic energy Wk FR of the accelerated plasma in the FRR equation (14) at the same time t. This positive disturbed energy compensates exactly the smooth negative energy in the wake.
As a result, the energy balance in the inflow region can be described as follows. While the FRR propagates along the current sheet and collects all plasma from the reconnected magnetic flux tube, the magnetic field is compressed in the narrow vertical layer above and below the FRR and moves as a compression wave. It turns out that although the disturbances of the magnetic field in the inflow region are sufficiently small (order of e ), nevertheless, the total energy in the compression wave is approximately twice as big as the kinetic energy of a plasma jet. One can say that the efficiency coefficient of the reconnection process is equal to 1/2 in the sense that the generation of the plasma jet which transport some amount of the kinetic energy needs the double depletion of the magnetic energy in the wake.
It is generally believed that the outflow region can transport along the current sheet not only energy and flux but also momentum. As was shown, the moving FFR itself does transport momentum (equations (17) and (18)). On the other hand, we saw that the small disturbances in the inflow region can essentially contribute to the energy balance, and therefore we have to investigate details of momentum balance in the similar way.
Earlier it was supposed that the momentum of the system is conserved because there are two FRRs which move in opposite direction. However, besides of this, there are the plasma flows in the inflow region which have to transport momentum as well. A huge quantity of plasma is contained in the inflow region, and although the velocity of this plasma is less than the plasma jet velocity, it can be expected that the momentum of the IR is comparable with FRRs momentum.
For the investigation of the plasma flows in the inflow region the stream function is introduced:
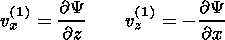 | (32) |
The Y function can be calculate by means of the Poisson integral in the inflow region as a whole:
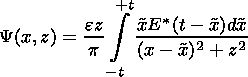 | (33) |

|
| Figure 4 |
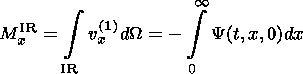 | (34) |
In this case the stream function on the x line may be calculated using the equation for the first order component of the plasma velocity at the boundary of the FRR (equation (11))
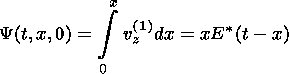 | (35) |
Now we can rewrite equation (34) as follows:
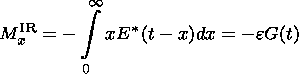 | (36) |
The expression under the integral is equal to zero everywhere except in the interval (x:[t-1,t]), that is, the region above the FRR. So, the part of the plasma in the vertical column above the FR region has only negative momentum. Bearing in mind that the momentum of the outflow region itself is equal to G(t) equation (18), it is clear that the momentum of the inverse flow in the IR exactly compensates the momentum of the plasma jet and this inverse flow is concentrated in the column above the FRR. Hence it turns out that the moving FR region together with the inverse flow outside does not transport any momentum at all. So, generally speaking, it is impossible to create a rocket using the reconnection process.

|
| Figure 5 |
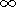 ))
)) 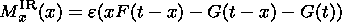 | (37) |
From the first, this function is zero in the wake of the FRR, then it begins to increase and after that it stays constant. From Figure 5 one can see that the behavior of the x component of the momentum of the plasma jets and of the inflow region is identical. So, in consequence to the inverse flow, the momentum of the structure which consists of the outflow region and the compression wave is equal to zero, and this mean that this complex object transforms no momentum.
In the case of the more realistic configurations of the initial magnetic fields [Kiendl et al., 1997; Rijnbeek et al., 1991] the energy and momentum balance are still similar to the simplest case.
|
| Figure 6 |
It should be emphasized that asymmetry decreases the amount of energy in the compression wave to a value of the kinetic energy of the plasma jets and changes the distribution of this energy between different parts of the wave. From the side of the bigger field the energy of magnetic disturbances is bigger than this energy from the other side.
A skew of the magnetic fluxes leads to increasing disturbed energy in the column above and below the FRRs. In this case, still more energy is contained in the compression wave, and therefore the loss of energy in the wake is more as well.
During the reconnection process the reconnection electric field, which is dissipative by nature, is transported through the current sheet via MHD waves and acts as a convective electric field there. As a result of that, a complex MHD object is formed. It consists of FRRs and special compression MHD wave, which transports no momentum and transfers both kinetic and magnetic energy.
Inside the field reversal region the magnetic energy is directly converted into kinetic energy of the plasma which is accelerated at the boundary of the FRR. The magnetic field reduction is balanced exactly by the kinetic plasma energy which is generated at the slow shock that can be expressed by the following relation:
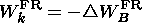 | (38) |
|
| Figure 7 |
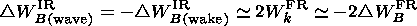 | (39) |
In summary, the total energy budget of an elementary reconnection event behaves as follows: Inside the outflow region, there is a deficit of magnetic energy compensated by enhanced kinetic energy, leading to accelerated plasma jets. Outside the FRR, magnetic energy is accumulated ahead of the FRR as a result of its fast motion, leaving a rarefaction wake behind. After the generation of the plasma jets with some amount of kinetic energy the deficit of the magnetic field energy in the region between two FRRs proves to be double that of the kinetic energy.
The momentum of the accelerated plasma inside the FRR is exactly balanced by the momentum of sufficiently slow plasma inside the column above the FRR in the inflow region, so that the total momentum of the structure which consist of the plasma jets and the compression wave, is equal zero.
So, a complex object is formed as a result of reconnection. It transports no momentum and transfers both kinetic and magnetic field energy.
Biernat, H. K., Reconnection at the days magnetopause: Theory and comparison with data, Trends Geophys. Res., 535, 1993.
Biernat, H. K., M. F. Heyn, and V. S. Semenov, Unsteady Petschek reconnection, J. Geophys. Res, 92, 3392, 1987.
Coroniti, F. V., Explosive tail reconnection: the growth and expansive phases of magnetospheric substorm, J. Geophys. Res., 90, 7427, 1985.
Furth, H. P., J. Killen, and M. N. Rosenbluth, Finite-resistivity instabilities of a sheet pinch, Phys. Fluids, 6, 459, 1963.
Heyn, M. F., and V. S. Semenov, Rapid Reconnection in compressible plasma, Phys. Plasmas, 3, 2725, 1996.
Hones, E. W., Magnetic Reconnection in Space and Laboratory Plasmas, Geophysical Monogr. Ser., vol. 30, AGU, Washington, D.C., 1984.
Kiendl, M. T., V. S. Semenov, I. V. Kubyshkin, H. K. Biernat, R. P. Rijnbeek, and B. P. Besser, MHD analysis of Petschek-type reconnection in non-uniform field and flow geometries, Space Sci. Rev., 79, 709, 1997.
Ottaviani, P. L., and G. P. Porcell, Fast nonlinear magnetic reconnection, Phys. Plasmas, 2, 4104, 1995.
Parker, E. N., The solar flare phenomenon and the theory of reconnection and annihilation of magnetic fields, Astrophys. J. Suppl. Ser., 8, 177, 1963.
Petschek, H. E., Magnetic field annihilation, NASA Spec. Publ., SP-50, pp. 425-439, 1964.
Priest, E. R., The magnetohydrodynamics of current sheets, Rep. Progr. Phys., 48, 955, 1985.
Rijnbeek, R. P., V. S. Semenov, A. A. Shmaltc, H. K. Biernat, M. F. Heyn, and B. P. Besser, Time-dependent reconnection in a current sheet with a velocity shear Planet. Space Sci., 39, 1377, 1991.
Semenov, V. S., M. F. Heyn, and I. V. Kubyshkin, Reconnection of magnetic field lines in a nonstationary case, Sov. Astron. (in Russian), 27, 660, 1985.
Semenov, V. S., I. V. Kubyshkin, V. V. Lebedeva, R. P. Rijnbeek, M. F. Heyn, H. K. Biernat, and C. J. Farrugia, A comparison and review of steady-state and time-varying reconnection, Planet. Space Sci., 40, 63, 1992.
Sweet, P. A., The neutral point theory of solar flares, in Electromagnetic Phenomena in Cosmic Physics, edited by B. Lehnert, pp. 123-129, Cambridge Univ. Press, New York, 1958.
Vasyliunas, V. M., Theoretical models of magnetic field line merging, Rev. Geophys., 13, 303, 1975.